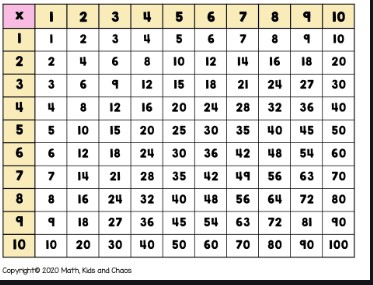
I was not a good student growing up. The three of my older siblings who preceded me in the same grade school made good grades, but not me. My grades in every subject was "S" which stands for "Satisfactory". I did not "apply myself". There was no doubt that my favorite subject was recess. My earliest math related memory was the time that my second-grade teacher smacked me with a yard stick for not paying attention when we were learning the multiplication table. I didn't care what six times eight was.
Next came junior high school, that was grades 7, 8, 9. Now we had letter grades. I don't think that I ever made an F, mostly C's, (we were graded every six weeks) but I don't remember making an A either except in one math class which I will tell about. No, I was still not "applying myself".
I think that it was in the 8th grade when I took a course which was probably called "beginning algebra". This was a course which caught my attention. I thought that it was kind of fun to solve equations. I could grasp everything and follow along with the teacher and the book. Everything was fine until one day the assistant principal showed up in class to get me and take me to his office.
He sat me down and told me that my teacher says that I am not paying attention in class. Good grief! How can my teacher say that I am not paying attention? But I figured out what was going on. Yes, I was not paying as much attention as the other students because I caught on to things quickly and did not have to spend two or three days going over the same kind of problems. She showed us how to solve a pair of linear equation with two unknowns. I saw how to do it, I did it and then I was ready to move on to something else. I guess I was just bored by the repetition and I really was not paying attention at times. But that was where I got my A and probably the only A I made in junior high.
And then there was the other math course. I think it was called "general math". It was awful. I remember doing things like adding up columns of figures and calculating percentages. There was business jargon and bookkeeping jargon and I was not at all interested. I did not do the homework and just got by with a passing grade.
About the same time that I started to high school, grades 10, 11 and 12, my older brother Milton was well along toward a degree in petroleum engineering at the University of Texas. I think that it was because I idolized my brother and wanted to succeed as well as he was doing, that I finally took a critical look at myself and decided to straighten up and start taking my schooling seriously. For the first time, I thought that I might need to go to college myself. I also did not want to spend my life working on windmills. Windmilling is another story. So ultimately, my grades were better in high school with all A's in math, physics, chemistry, and mechanical drawing. Oh yeah, I made A's in physical education and graduated as the outstanding student in my graduating class in physical education. That was a surprise!
But this story is about my mathematics upbringing. So, I must tell about my 10th grade algebra class. The teacher(?) was the husband of the high school Spanish teacher. Maybe that was how he got the job. The administration must have been desperate to fill the position because they hired someone to teach algebra who knew nothing about the subject. He would assign problems from the book and send students to the board to work them on the board. He never worked a problem himself because, I think, that he did not know how. Most of our class time was spent by just talking. He had his favorite students who would talk to him on any subject. Those students sat on one side of the room and I was on the other. What I wanted was to learn algebra and I did! I read the book and went over the examples and worked all the problems. I taught myself algebra.

I remember what happened one day: He usually only asked his favorite students on the other side of the room to go to the board. We were working some "word problems". You know, "a train leaves Chicago going west at 2:00 and another train …". He asked for someone to put this problem on the board and my hand was the only one to go up. I put it up there and explained my work. When I finished, he looked in the back of the book to see if I had the right answer.
Now my teacher gave an exam at the end of six weeks. I scored a perfect 100 on the exam and we got our six weeks grades a few days later. My grade was a B. What the Hell? He was sitting at his desk at the end of class with his grade book in front of him. I approached and asked him why I made a B. To my amazement, he asked me "What is your name?" I told him my name, he opened the grade book and there was my name with a 100 in the grade column and a B written next to it. He erased the B and wrote in an A.
Of course, I also had plane geometry too. The elderly teacher was very good. I thoroughly enjoyed proving the theorems and she loved it when the students succeeded in doing so.
Why do I tell the story of the incompetent algebra teacher? It was an odd experience for sure but I came out of it pleased that I was capable of learning mathematics and learning it on my own. At that point in my life I was sure that I would need algebra (and mechanical drawing) to get into the University of Texas like my brother did. I had good teachers in high school, especially in physics, chemistry and senior level English. But most of all, that experience of learning on my own initiative stuck with me. I think that it kind of prepared me for the type of teaching that I was privileged to have years later at the University of Texas; teaching without lectures, teaching which encouraged me to find the mathematical connections on my own instead of being shown everything by the teacher.
In 1998, I was interviewed by a gentleman who was writing a dissertation about the teaching of R. L. Moore. As we sat in my office, I began to tell him about my experience when I took Math688, Moore's first topology course. I told him about how I was overwhelmed at first and then during the Christmas break, I finally caught on and made a big comeback. As I told the story, I became very emotional and there were even tears in my eyes before I finished telling my tale.
I realized then how much the story meant to me as a defining time in my life. My story was also a tribute to the teaching method of R. L. Moore. After the interview, I went home, sat down at my computer, and wrote Christmas in Big Lake. I gave a copy to the interviewer the next day. He passed it on to the Educational Advancement Foundation, an organization which was originally dedicated to the teaching method of R. L. Moore. My story was a popular handout at their meetings and is posted on their web site. Other organizations have asked for my permission to place a copy on their web site and it has been excerpted in at least four books.
Christmas in Big Lake
It was the Spring of 1959 and I had struggled that school year to catch on to the theorem proving courses of Fitzpatrick and Wall. It was coming together for me to some extent although I was not doing nearly as well as many of the other students such as my friend Harvy Baker, for example. I do remember that I managed to give an epsilon - delta proof of the fundamental theorem of calculus. But Harvy came up with a description of a set of real numbers which we now know as the Cantor set and I thought that there was no such thing. I tell you this so you will know about where I stood in my newly chosen major of mathematics.
By that Spring, Harvy and I and other classmates had heard stories about R. L. Moore and his famous Math688 course. We were curious to find out if we could or should sign up for it the following Fall semester. We were undergraduates and would have to get special permission and we were not sure it was the right thing to do. So we went to talk to Ben Fitzpatrick and asked for his advise. I remember that Ben warned us that it would be very different from anything we had experienced before. And I guess he gave us the advice of going ahead and signing up because in September we were there.
Dr. Moore began the course by stating Axiom 0 (Every region is a point set) and some definitions and the first few theorems. The undefined terms were "region" and "point". On the second day of class Dr. Moore asked who could prove Theorem 1. Actually, he may have started down the class roll and asked students one at a time if they could prove Theorem 1. There were plenty of students who could prove the first few theorems, which were elementary consequences of the definitions. But I did not understand how to prove the theorems and I did not understand the proofs that were given. Looking back on it now, I think that I failed to understand that the theorems were independent of the meaning of the undefined terms. I was laboring under the assumption that the students who went to the blackboard to present a proof knew more about "regions" than I did.
I was confused about some other things too but it does not matter why, I was simply baffled and flustered by the entire process. I continued to take notes meticulously and this habit turned out to be a contributor to my eventual salvation. I would write down the words of a proof and copy the drawings that students were putting on the board and then I would copy it all again neatly into a spiral notebook. After a few weeks we got to some of the more difficult theorems, or so it seemed to the class. To me, they were all impossible. I continued to copy the proofs which were just so much gibberish to me.

Dr. Moore's (left) method of calling on students for proofs involved calling on the persons first who had contributed the least. My name rose to the top of the list. The class met at 9:00 AM on Tuesday, Thursday and Saturday and my day would usually begin the same way. Dr. Moore would take his place in front of the classroom and might make a few comments or remind us of where we were in the material yet to be done. Then he would look at me and ask the same question, "Mister Young, can you prove the next theorem?". It was a bit terrifying and humiliating but I felt no negative feelings toward him. After all, I knew how to extract myself from the situation. I had to prove a theorem and get the pressure off of myself. But my answer was always the same, "No sir, I cannot". It sounds cruel as I describe it now but there were no hurt feelings. It was as much comical as cruel. Someone had to be the worst student in class and it was me.
In those days, the Fall semester did not end until after the Christmas break. Our class would meet three or four times after we got back from Christmas. On the last class meeting before Christmas, Dr. Moore spent the entire hour stating theorems and definitions. He really loaded us up with work to do. I took my notes and went to be with my parents for Christmas. My parents had moved that year from San Angelo to Big Lake, Texas, a small oil town 80 miles to the west. They lived in a metal building which had been converted from the office of a welding shop into living quarters. I knew practically no one in the town and so I was as isolated and undistracted as I could possibly be for the task before me. I believe that I began as usual to make good copies of the latest proofs that were given in class.

This time, as I read the proof of Theorem 25 (I am not sure if 25 is the number) that my classmate had presented, I somehow managed to understand the argument. I saw how he had made use of a previous theorem and the definitions which were involved. I was astonished! I was understanding one of the proofs for the first time. I went to the proof of Theorem 24 and I managed to understand that one too. I remember thinking that perhaps I could prove some of those theorems myself. I worked my way back through the theorem sequence and proved almost all of the theorems in my own way and wrote my own proofs in my spiral notebook including those very elementary theorems at the beginning. I worked all day every day through Christmas doing this. Finally, with a few days left before heading back to Austin, I was ready to look at the work that Dr. Moore had given us to do during the break.
I wish that I had the ability to describe the exhilaration that I felt to the core of my existence when I put together a proof of Theorem 26. I marched forward proving the next theorem and the next. I am not saying that it was easy, it was exhausting work. I would get up early in the morning and work all day. I wonder what my mother thought about all this. She probably thought that her son who went off to college had to study. She could not have known that what I was doing went well beyond studying. She could not have known that her son became a mathematician that Christmas.

The time came to go back to Austin and a great fear came over me as I visualized the inevitable scene that would unfold in the classroom. I was ready and confident with proofs of several of the upcoming theorems but I was scared to death. I had never "been to the board". When the time came, Dr. Moore looked at me and asked the usual "Mister Young, can you prove Theorem 26?". I am pretty sure that my exact answer was "yes sir, I can". I had learned that he did not like for anyone to say "yes, I think I can". The fear that I felt at that moment is indescribable but I want to repeat that I was confident that my proof was correct. The fact that I knew I had it, the fact that I was confident in spite of the awful fear of the moment is a tribute to the Moore method of teaching. I went to the board and presented my proof. He asked if anyone had any questions. There were none and I took my seat.
My friends who have had courses from Dr. Moore are always surprised when I tell them what happened next. He never called upon the same person who had just presented a proof. It was just not done. He would turn the pressure on someone else. But I distinctly remember that he looked around the room as if trying to decide whom to call upon. His eyes fell upon me again and he asked "Mister Young, can you prove Theorem 27?" I said that I could and went to the board again. When I finished I sat down again and again he looked around pretending to decide and said "Mister Young, can you prove Theorem 28?". This impish routine continued for an hour and started again at the next class meeting. In fact, no one else was called upon for the remainder of the term. I proved all of the theorems that we had time for.
Unfortunately, I was not able to continue with my class that next semester because of a conflict with the only section of Russian that was offered and I had to have the Russian class to graduate. I resumed the MH688 course the next year as a graduate student and took the MH689 course the year after that. I also took Dr. Moore's measure theory course. Along the way I became a student of H. S. Wall and chose that route for my PhD work. I will always remember that Christmas in Big Lake and the feeling of triumph that resulted - a measure of success for the Moore teaching method and success for myself which propelled me toward a career in mathematics. Of course, I will always treasure the influence that he had upon me. I like to think that maybe, just maybe, he never forgot me either.
Sam Wayne Young, Opelika, AL, March, 5, 1998
If you have read The Early Years, you know that I said that I was headed for a degree in Engineering. After high school, I had two years in a junior college preparing me to major in Petroleum Engineering. Then, I entered the Engineering School at U. T. in 1957. I spent a year in the School of Engineering and then what happened? My story, The Lost Envelope will answer that question and is a prequel to Christmas in Big Lake.
The Lost Envelope
In the spring of 1958 toward the end of my first year at the University of Texas, I was contacted by Continental Oil Company. Their representatives were on campus and wanted to talk to me. I was delighted to hear this, maybe they will tell me where I will be working this summer, I thought. I was designated as a trainee for Conoco. I worked for them in the summer to earn enough to go to college and I was to be a petroleum engineer for them when I graduated. At least that was the way I thought it was as I went for my interview. To my astonishment, they fired me, dropped me from the trainee program without warning. It was late in the year and I did not have a summer job without which I could not continue in college. I was broke and angry.
My anger was directed toward the entire oil industry and toward the Engineering School. I had endured at least two very bad experiences with engineering teachers during the year and I would like to include a description of those events because this story is partly about teaching and how I accidentally found the best that I possibly could have found.
In one case, a petroleum engineering teacher posed a problem on an exam the premise of which was impossible. It was not that he made the mistake in composing the problem that troubled me. Instead, when one of my classmates and I and even his own office mate teamed up to convince the teacher of his error, he did not have the intellectual honesty to admit the error to the class. Instead, he conducted a sort of pitiful cover-up and treated my collaborating classmate and I very poorly after that. It was clear that his pride was more important to him than the accuracy of mathematical calculations based upon elementary physics.
Another incident involved a problem on a fluid mechanics exam which was worth 20 points out of 100 points. I was sure that I had solved the problem correctly but to my amazement, I was awarded 0 points for my efforts. In the process of solving the problem, one is required to set up a simple differential equation and solve it. Of course, I do not remember now what the equation was but it must have been something very simple because I had not had a course in differential equations. I knew my derivatives and integrals and I just solved it by observation. The teacher told me that indeed I did get the right answer but he is giving me a 0 because I did not demonstrate the correct method for solving the differential equation. His explanation included the strong implication that I had cheated! Our relationship was poisoned from that point but I was the one who was cheated- cheated out of a chance to have an understanding teacher (and cheated out of 20 points too).
So, there I was in the spring of 1958 with no prospects of a job to make enough money to continue in college and worst of all, no ambition at all to continue in my chosen field of engineering. After all, how much respect could I have for my teachers? Were they to be so befuddled by the mathematical accomplishments of someone who had two semesters of calculus at a junior college?
I do not remember too much about what I did that summer. I hitchhiked between Austin and San Angelo, collected some unemployment checks and caught some odd jobs putting together just enough money to enroll for fall semester. That was all - just enough to enroll. I later got a job grading homework papers for $60 per month. The credibility of this entire tale will be jeopardized when I tell you that I managed to live on $60 per month that year- I did. But, on with the story. One of my friends was a mathematics major. Before I met him, I did not know that Universities award degrees in mathematics. Knowing that I probably would not have the money to make it through the semester anyway I thought - why not be a mathematics major?
** For the remainder of this story I will use names and places without explanation or apology to those unfamiliar with same. **
I found out where the Mathematics Department was and found my way down to Benedict Hall to see an advisor. They directed me down the hall to the office of R. E. Greenwood. I sat down with Dr. Greenwood and he told me that I would have to take two years of a foreign language and so I should sign up for a foreign language, advanced calculus, differential equations, and an elective. Simple enough, but he jotted these things down on the back of an envelope including, (this is important to note), the course numbers. I took the envelope to my place and put it somewhere in my desk.
Registration at U. T. in those days was a week of total madness in hot Gregory Gym. Registration times were assigned by last names and my hometown friend and I were scheduled the same day and hour. We drove to Austin from San Angelo that morning arriving just in time to grab our materials and head for the gym. I looked for the envelope and could not find it. We were in a hurry and rather than take a chance on making us both late, I gave up looking for the envelope. After all, I knew what to sign up for; Russian, advanced calculus, differential equations and advanced strength of materials.
Some people believe in "fate" or some form of "divine guidance". I can not subscribe to such beliefs because, among other things, I have noticed that the divinity always gets the credit for the things that turn out for the better and none of the blame for the things that do not. I choose instead to take a less gracious view of reality. I believe in the theory of parallel universes. The only universe we are aware of is the one in which events have occurred exactly as our records show. We do not live in the universe in which I found the envelope that day. We live in the one in which I did not find the envelope that day. I am sure that everyone can point to an event in their own lives where a major bifurcation took place. Fine, we live in that universe too. For the sake of rationality, we should remember the bad along with the good, but the nice things are much more fun to recall. That is what I am doing here, indulging in the nice things.


There was no problem that I recall, getting the courses I wanted even though I did not have the course numbers with me. I signed up for beginning Russian and my engineering course elective and then I must have looked at a list of sections of mathematics courses, a list arranged in increasing numerical order. I simply scanned down the list until I came to the first section that was open that was labeled "advanced calculus". I looked down the list to the first open section that was labeled "differential equations". The thing that is important to point out here is that Mh321 comes higher on the list than Mh325 which is also advanced calculus and Mh322 comes before Mh326 and so it was Mh321 and Mh322 that I signed up for.

On the first day, I reported to my math classes which were both on the third floor of Benedict Hall. My advanced calculus teacher was a fresh PhD by the name of Ben Fitzpatrick (lower left). My differential equations teacher was a white-haired gentleman by the name of H. S. Wall (upper left). The burden of these two unlucky teachers was just beginning as I struggled with totally mysterious epsilon-delta definitions in one class and horizontal and vertical line definitions in the other. It will suffice to say here that the unbounded patience of both teachers eventually bore some fruit before the year was over.
Meanwhile, at the place where I lived, my friends were all puzzled to know how in the world I managed to sign up for the wrong courses. They all knew about "2nd floor" courses and "3rd floor" courses but no one ever told me. As the year progressed, however, it became clear to me that I was on the right floor of Benedict Hall after all and the business of the lost envelope was completely forgotten. I do not know how much longer afterwards it was when it happened. I was probably cleaning out my desk when I came across a business size envelope. On it was written in someone else's (Dr. Greenwood's) hand; foreign language, elective, advanced calculus MH325, differential equations MH326. I had not followed the advice of my advisor.
Seven years after I blundered into his class, Dr. Wall placed his name on my PhD dissertation, a dissertation which he had directed. The dissertation was also signed by Drs' R. L. Moore, R. G. Lubben, and R. E. Greenwood. These were four of the teachers who pushed me to do my best and never, never disappointed. My acquaintance with Ben Fitzpatrick continues to this day. He has been my teacher, my friend, my colleague, and my source of guidance and inspiration for all of these years. Anyone who knows Ben can tell you how fortunate I am to have had the opportunity to be influenced by him in my life and in my career. It is on the occasion of Ben Fitzpatrick's retirement from Auburn University that this note is written.
Sam W. Young May 27, 1997 Opelika, AL
Upon my completion of the PhD at the University of Texas in 1965, my career as a mathematics professor began at the University of Utah in 1965. Diane finished her PhD at Utah and we moved on from there. I retired from the faculty of Auburn University in 1997 after working there for 28 years. One day, towards the end of my time at Auburn, I was talking with two of my math friends, colleagues about our early years and why and how we wound up as mathematicians. The question was "Why did you do it?" I do not remember what my friends' answers were but I remember what my answer was. I said that "I wanted to work indoors". I refer you to my tales about windmilling with my father.
Yes, I was my father's helper working on windmills during the Summer and Christmas breaks. This was the case until the end of my second year in junior college when I landed a job working in the oil field. Going back to school in the Fall was always a blessed escape from windmills. And school was, after all, indoors. My first tale of my beginnings in mathematics is Math-The Early Years. I put this together recently.